what is the method used to find the equation of the regression line called

Regression Line Formula (Table of Contents)

• Formula
• Examples

What is the Regression Line Formula?

The term "Regression Line" refers to the statistical technique which is used to model the human relationship between ii variables. In this technique, there is an explanatory variable and a dependent variable, and the former is used to draw insights about the latter and build its forecast. In other words, it is used to brand predictions nigh the dependent variable based on its relationship with the explanatory variable. The formula for the regression line (Y) tin be derived past multiplying the slope of line (b) with the explanatory variable (Ten) and then adding the result to the intercept (a). Mathematically, the regression line equation is represented as,

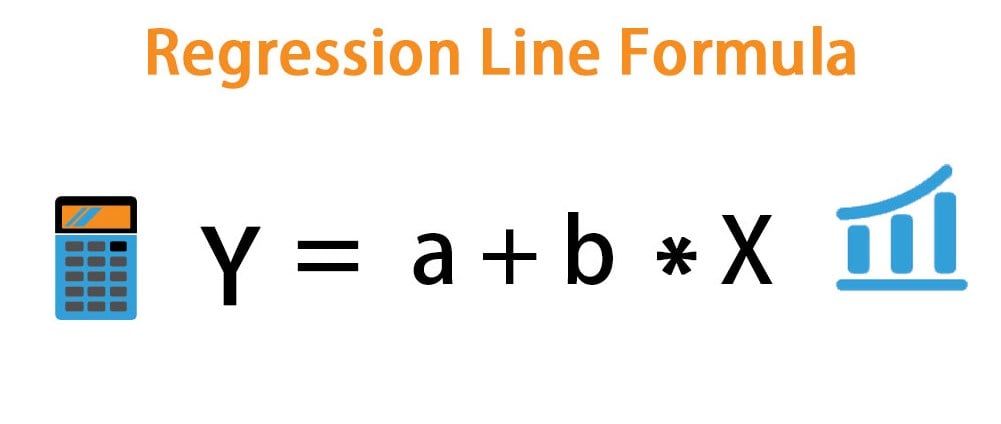
The formula for Regression Line –

Y = a + b * X

Example of Regression Line Formula (With Excel Template)

Let's take an instance to understand the calculation of the Regression Line in a better manner:

You lot tin download this Regression Line Formula Excel Template here – Regression Line Formula Excel Template

Case #1

Let united states accept the example of a set of v patients whose glucose levels take been examined and presented along with their corresponding ages. Based on the given data, build the regression line equation and and then calculate the glucose level for a person aged 77 by using the regression line equation.

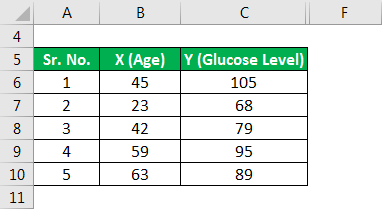
Solution:

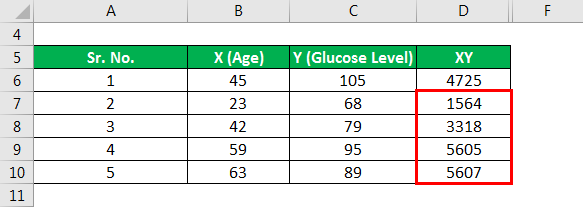
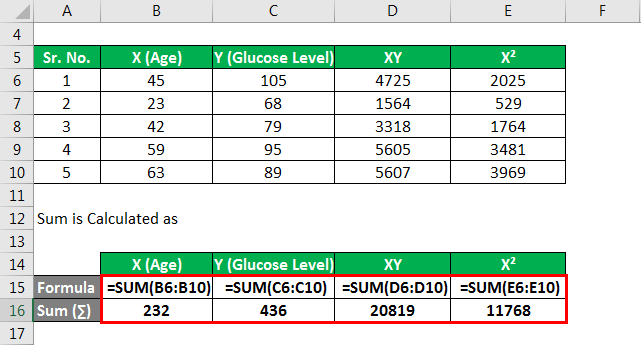
Now let's calculate XY for ΣXY.

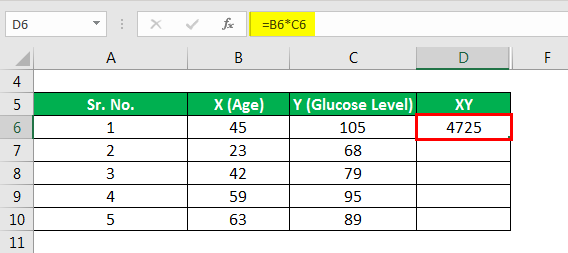
Similarly, calculated the below XY.

Now let's summate X² for ΣX².

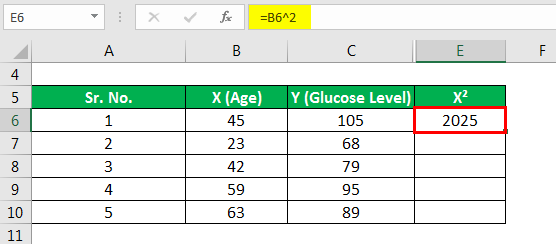
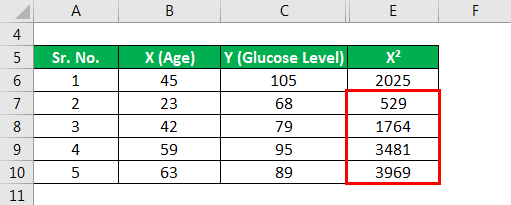
Similarly, calculated the below X².

Sum is Calculated as

b is calculated using the formula given below

b = [n * (∑XY) – (∑X) * (∑Y)] / [north * (∑X^two) – (∑X)²]

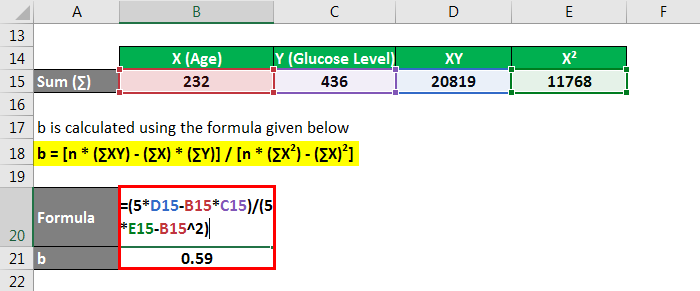
• b = [5 * 20819 – 232 * 436] / [5 * 11768 – (232)ⁱⁱ]
• b = 0.59

a is calculated using the formula given below

a = [(∑Y) * (∑X²) – (∑X) * (∑XY)] / [due north * (∑Ten^two) – (∑Ten)²]

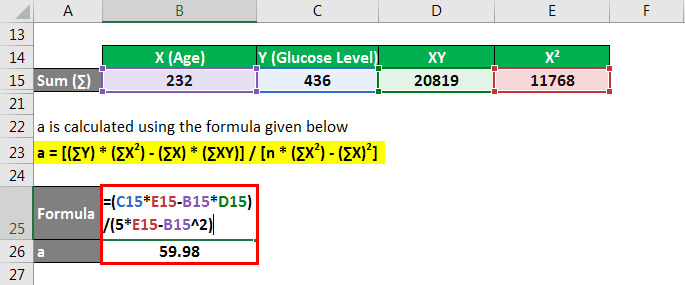
• a = [436 * 11768 – 232 * 20819] / [5 * 11768 – (232)²]
• a = 59.98

In the above equation, the glucose level of a person aged 77 years can be calculated equally,

Regression Line is calculated using the formula given below

Regression Line Formula = Y = a + b * X

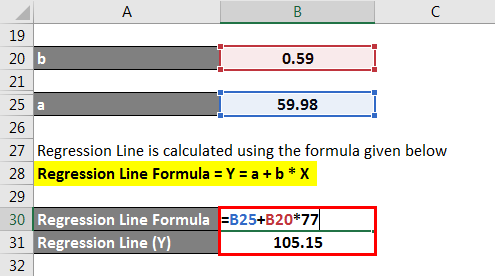
• Y = 59.98 + 0.59 * X
• Y  = 105.fifteen ~ 105

Therefore, equally per the regression level, the glucose level of a 77-year-former person is predicted to be 105mg/dL.

Example #2

Let us take the case of a class with 10 students where their heights and weights were measured to check if their weight had whatsoever liner relationship with their height. The post-obit data is available.

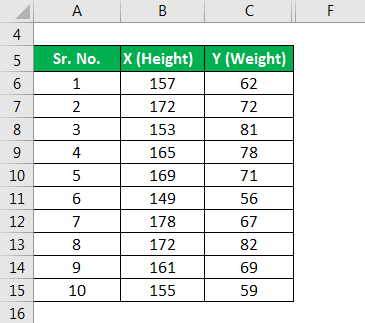
Solution:

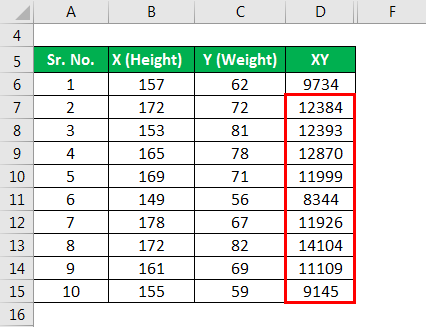
Now let's calculate XY for ΣXY.

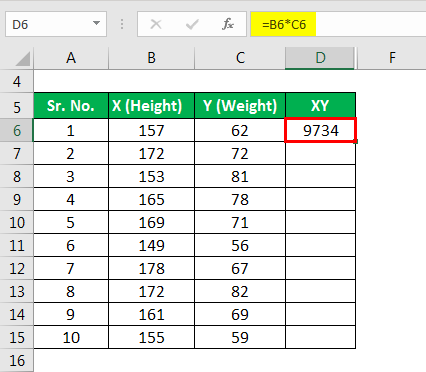
Similarly, calculated the below XY.

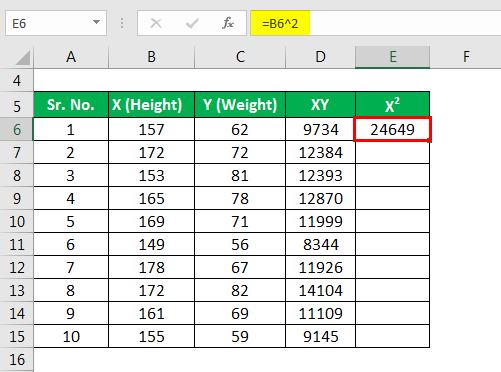
Now permit's calculate X² for ΣX².

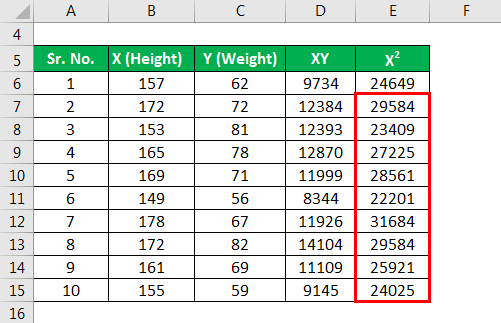
Similarly, calculated the below X².

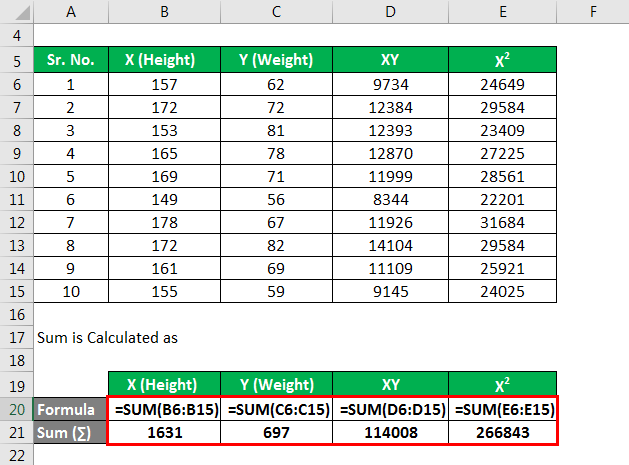
Sum is calculated as

b is calculated using the formula given below build the regression line equation based on the given information.

b = [due north * (∑XY) – (∑X) * (∑Y)] / [n * (∑X²) – (∑X)²]

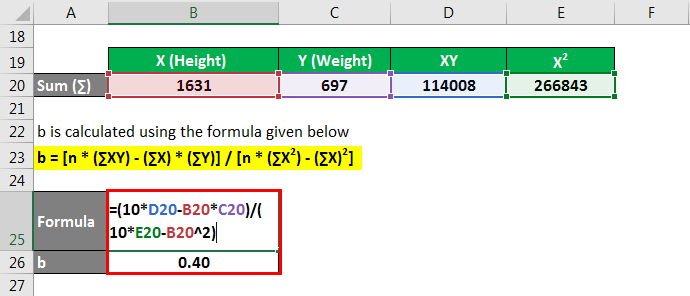
• b = [x * 114008 – 1631 * 697] / [10 * 266843 – (1631)ⁱⁱ]
• b = 0.xl

a is calculated using the formula given below

a = [(∑Y) * (∑Tenⁱⁱ) – (∑X) * (∑XY)] / [north * (∑X^two) – (∑X)²]

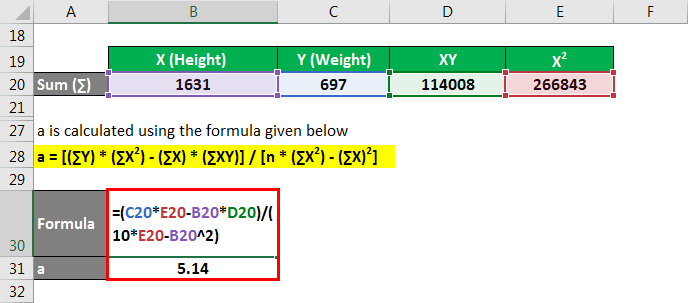
• a = [697 * 266843 – 1631 * 114008] / [10 * 266843 – (1631)²]
• a = 5.14

Regression Line Equation is calculated using the formula given beneath

Regression Line Formula = Y = a + b * X

Y = a + b * X

Or Y = 5.14 + 0.twoscore * X

Explanation

The Regression Line Formula can be calculated by using the following steps:

Footstep i: Firstly, determine the dependent variable or the variable that is the subject of prediction. It is denoted by Y_i.

Stride 2: Adjacent, make up one's mind the explanatory or independent variable for the regression line that Xi denotes. It should exist selected such that it can fairly explain the variation in the dependent variable.

Step 3: Next, make up one's mind the slope of the line that describes the relationship between the independent and the dependent variable. It is denoted by "b" and is calculated based on the number of data points (n), explanatory and dependent variable past using the following formula

b = [north * (∑XY) – (∑X) * (∑Y)] / [n * (∑Xⁱⁱ) – (∑Ten)ⁱⁱ]

Stride 4: Side by side, make up one's mind the intercept of the regression line that remains constant irrespective of the explanatory variable's value. It is denoted by "a" and is calculated based on the number of data points (due north), explanatory and dependent variable past using the post-obit formula

a = [(∑Y) * (∑10²) – (∑X) * (∑XY)] / [n * (∑X²) – (∑X)²]

Step v: Finally, the formula for the regression line can be derived by multiplying the explanatory variable (step ii) and the slope of the line (step three) and then adding the result to the intercept (footstep iv) as shown below.

Y = a + b * X

Relevance and Apply of Regression Line Formula

It is one of the few important concepts for model edifice, and information technology is predominantly used to build the predictive model by applying the technique of best fit to the relationship between explanatory and dependent variables. Information technology finds applications in various finance models that include the CAPM method, revenue forecasting, etc.

Recommended Articles

This is a guide to the Regression Line Formula. Here we discuss how to calculate the Regression Line along with practical examples. We likewise provide a Regression Line calculator with a downloadable excel template. You may also look at the following articles to learn more than –

1. Examples in Depreciation Formula
2. Calculations in Convexity Formula
3. How to Calculate Internet Exports?
4. What is the Moving Average Formula?

davisforew1997.blogspot.com

Source: https://www.educba.com/regression-line-formula/

Share this post